Proofs:

Note to Angle Sigma:

***************

Proof 1:  Angle Sigma

***************

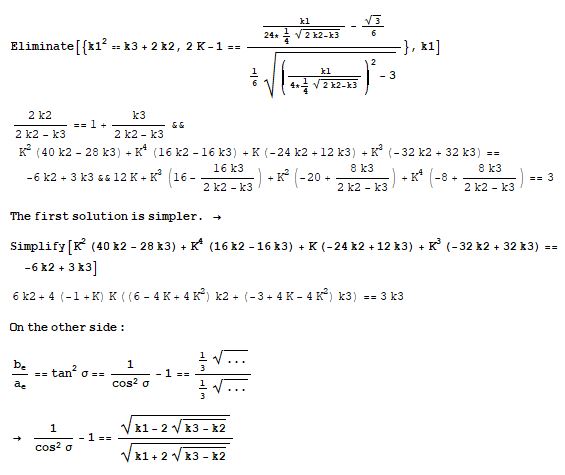
Proof 2: Collinearity of points Pa, Pb, Pc and G

This proof is independent of angle φ = φa = φb = φc (cf. Fig. 08 of the website)

and is therefore also valid for the theorem with  common tangents to the McCay circles!

********************************************

Proof 3: After the Affine Transformation the Angle  ε = 45°

***************

Proof 4: 

***************

Proof 5: 

********************************************

Proof 6:

... that the c-McCay circle is also the G-Apollonius circle of line segment McCbr:

... see proof 3.

[back]